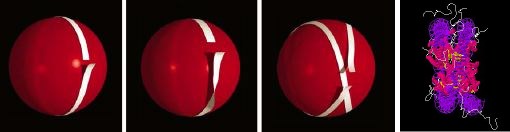
1. A singly encircled band with two half-twists is topologically equivalent to a doubly-encircled untwisted band.
2. The Linking number describes both forms, and comprises;
   Lk = T_w + W_r
   W_r or Writhe measures the extent to which coiling of the central curve has relieved local twisting T of the ribbon (T_w is ∫T)
3. Writhe connects the manifold of a single doubly-twisted band in 2D space (T=2, W=0) with that of a doubly-encircled untwisted band in 2D space (T=0, W=2) by projection into 3D space (T + W = 2)
• Positive values of W_r are termed overtwisting by supercoiling
• Negative values of W_r are termed undertwisting by supercoiling
4. Both T_w and W_r are signed Chiral indices.

The White, Cãlugãreanu, Fuller Theorem; J. H. White, Am. J. Math., 1969, 91, 693-728; G. Cãlugãreanu, Czech Mathematics J., 1961, 11, 588-625; F. Fuller, Proc. Natl. Acad. Sci., 1971, 68, 815-819.